Subsections

• Principle of Maximal Entropy
• Reversibility Paradox
• Temperature
• Sign of Temperature

Entropy and Probabilities

Principle of Maximal Entropy

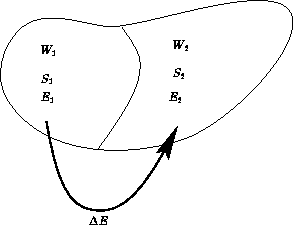
Two macroscopic states A and B. Suppose S(A)>S(B). What can we say about probabilities?

All microscopic states in microcanonical ensemble are equal. Probability is proportional to the number of states.

$$\frac{\Prob(B)}{\Prob(A)} = \exp\bigl(S(B)-S(A)\bigr)<1$$

The state with greater entropy is more probable

Thermodynamic limit: $S(A)\sim V$, $S(B)\sim V$, $S(B) - S(A)\sim-V$

$$\frac{\Prob(B)}{\Prob(A)}\to0, \quad V\to\infty$$

In thermodynamic limit we can see only the states with maximal entropy .

Closed systems:

• in equilibrium: $S=S_{\max}$
• out of equilibrium: $S<S_{\max}$

If we look at a closed system, it will be either in equilibrium, or equilibrating.

Consequence:

In all processes in closed systems entropy never decreases (Clausius-Boltzmann).

Reversible processes:

$S=\mathit{const}$

Irreversible processes:

$S\uparrow$

Reversibility Paradox

Equations of mechanics are time reversible . How can irreversibility appear in the picture?

Glass falls from a table an is broken. All molecular motions are reversible. What about irreversibility??

Answer:

Entropy is not a property of a microscopic state, it is a property of ensemble . Irreversibility is in our macro scopic eye!

Broken glass--many states, non-broken glass--one state.

Happy families are all alike; every unhappy family is unhappy in its own way.

Temperature

Definition:

Temperature is the inverse of the derivative of entropy by energy:

$$\frac{\partial S}{\partial E} = \frac1T$$

Theorem:

In equilibrium all parts of a closed system have the same temperature.

Proof:

Consider a reversible process in a closed system:

Change of entropy: $\Delta S = 0$

$$S_1(E_1) + S_2(E_2) = S_1(E_1-\Delta E) + S_2(E_2+\Delta E)$$

or

$$\frac{\partial S_1}{\partial E} = \frac{\partial S_2}{\partial E}$$

Sign of Temperature

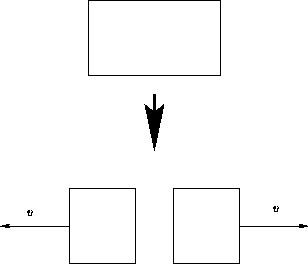
We discussed systems that do not move. What happens if it moves with the velocity v?

Entropy depends only on the internal energy:

$$E_{\text{internal}} = E - \frac{mv^2}{2}, S= S\left(E_{\text{internal}}\right)$$

What happens if a system separates into two parts that move apart?

$$E=\mathit{const},\quad E_{\text{internal}}\downarrow$$

If T<0, entropy increases --the process is favorable. Systems with negative temperatures would want to blast off!

In fully equilibrated systems T>0. But partially equilibrated systems might have negative T for some degrees of freedom.